2.
假設\([\; ]\;\)為高斯記號(說明:例如\([\;a ]\;\)表示小於或等於實數\(a\)的最大整數),請求出方程式\(x^2-12[\;x ]\;+11=0\)的所有解
。
[]表高斯符號,求解\(3x^2-19 \cdot [\;x ]\;+20=0\)。
(105高雄餐旅大學附屬高中,
https://math.pro/db/thread-2527-1-1.html)
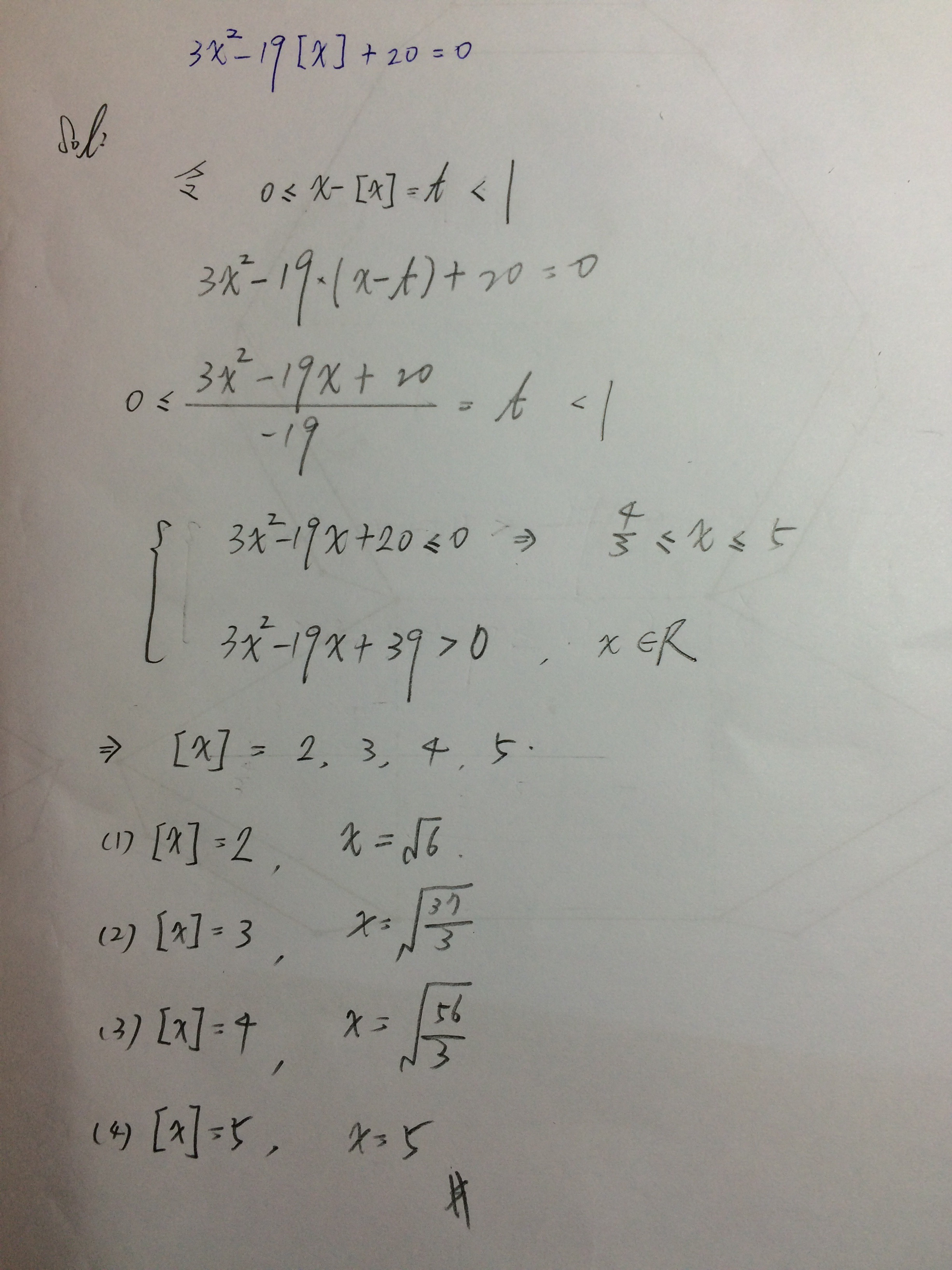
3.
設\(\alpha\)、\(\beta\)、\(\gamma\)、\(\delta\)為方程式\(x^4+13x^3+17x^2+6x+1=0\)的四個根,求\(\displaystyle \frac{1}{\alpha^2}+\frac{1}{\beta^2}+\frac{1}{\gamma^2}+\frac{1}{\delta^2}\)的值為
。
[提示]
令\(1+13y+17y^2+6y^3+y^4=0\)四根為\(\displaystyle \frac{1}{\alpha},\frac{1}{\beta},\frac{1}{\gamma},\frac{1}{\delta}\)
再用
https://math.pro/db/viewthread.php?tid=1019&page=1#pid2501方法下去算
設\( 4x^3+3x^2+2x+1=0 \)三根為\( \alpha,\beta,\gamma \),則\( \displaystyle \frac{1}{\alpha^5}+\frac{1}{\beta^5}+\frac{1}{\gamma^5} \)?
(100苑裡高中,
https://math.pro/db/viewthread.php?tid=1178&page=1#pid3963)
5.
設地球為空間中一球體。今以地球球心為原點,地球半徑為單位長,建立一個直角坐標系。若地球表面上有甲、乙、丙三地,甲、乙的坐標分別為\((1,0,0)\)及\(\displaystyle (0,\frac{1}{2},\frac{\sqrt{3}}{2})\),而丙地位於甲乙兩地之間最短的路徑上,且甲丙路徑長為乙丙路徑長的2倍,求丙地的坐標
。
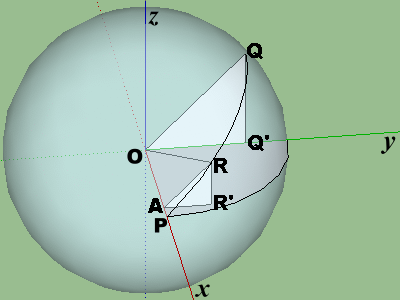
今一單位球(半徑為1的球)球心為原點,且球面上兩點P、Q座標分別為\( P(1,0,0) \)、\( \displaystyle Q(0,\frac{\sqrt{2}}{2},\frac{\sqrt{2}}{2}) \),延著球面行進,於PQ最短路徑中取一點R,使得(PR弧長):(QR弧長)=1:2,試求R點座標。
(99大安高工,
https://math.pro/db/viewthread.php?tid=960&page=1#pid2178)
12.
桃園高中80周年慶,師生想利用8個8組成一個校運昌隆數作為紀念,經過討論後決定以\(8888^{8888}\)作為此校運昌隆數。將此校運昌隆數展開後的各位數字和令為\(A\),再將\(A\)的各位數字和令為\(B\),求\(B\)的各位數字和為
。
When \(4444^{4444}\) is written in decimal notation, the sum of its digits is \(A\). Let \(B\) be the sum of the digits of \(A\). Find the sum of the digits of \(B\). (\(A\) and \(B\) are written in decimal notation.)
1975IMO,
https://artofproblemsolving.com/ ... _Problems/Problem_4
weiye解題,
https://math.pro/db/viewthread.php?tid=1387&page=1#pid6086
計算2.
已知\( abc=1\),\(a,b,c\)皆正,求證:\(\displaystyle \frac{1}{a^3(b+c)}+\frac{1}{b^3(a+c)}+\frac{1}{c^3(a+b)}\ge \frac{\frac{1}{a}+\frac{1}{b}+\frac{1}{c}}{2}\)
Let \(a, b, c\) be positive real numbers such that \(abc=1\). Prove that \(\displaystyle \frac{1}{a^3(b+c)}+\frac{1}{b^3(a+c)}+\frac{1}{c^3(a+b)}\ge \frac{3}{2}.\)
1995IMO,
https://artofproblemsolving.com/ ... _Problems/Problem_2